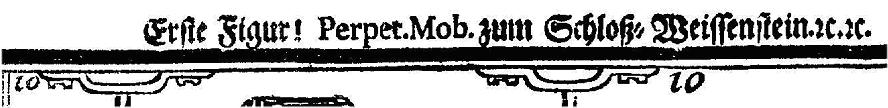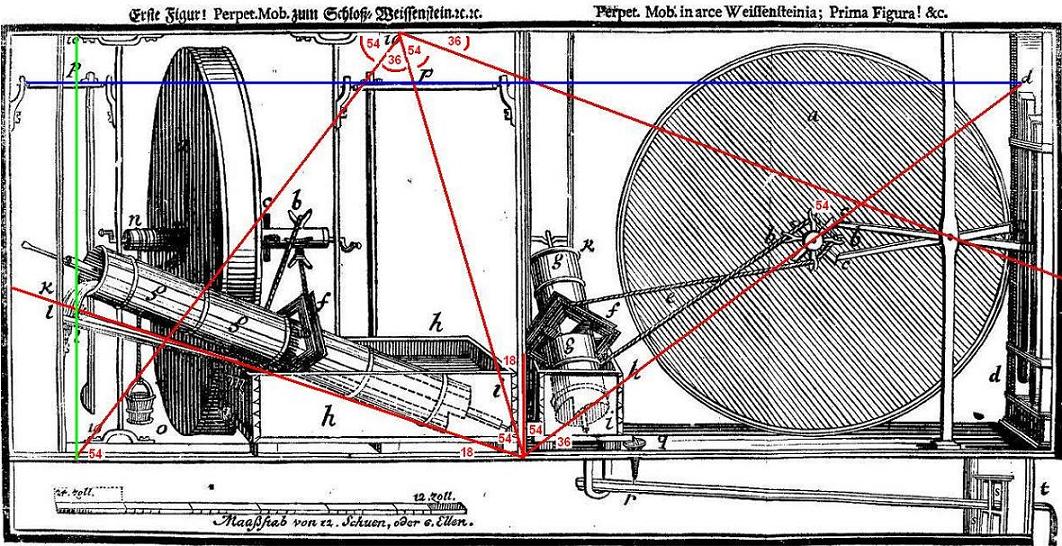
Weissenstein Castle water wheel

The above picture is from Bessler’s Das Triumphirende and shows how his wheel could be used to drive a hydraulic Archimedes pump. Those who are familiar with Bessler’s codes as diagnosed on these pages will not be surprised to observe that he seems to have over-labelled this drawing too. This is the term I use to refer to the inventor’s habit of labelling everything in his drawings not once but as many as four times but with certain inconsistencies. This system allowed him to introduce enough numbers which when added together produced a meaningful total, which always revealed the angles of the pentagram. The above seems at first sight to be a typical example.

One of the differences between this drawing and his previous ones is the fact that in this one the parts are labelled with letters rather than numbers. However there is one labelled part which is strangely ambiguous and that is the main supporting column which supports the wheel. It looks like a ‘W’ however it can also be taken for the number ten, but this cannot be right because the other parts are labelled with letters. A clue to this anomaly lies in the attached list of labelled parts; here the list is entirely ‘numbered’ in letters except for the last item which is definitely labelled and printed as a ‘10’. You can see the ambiguity in the expanded detail below, which has two examples of the number ‘10’, or is it the letter ‘W’?

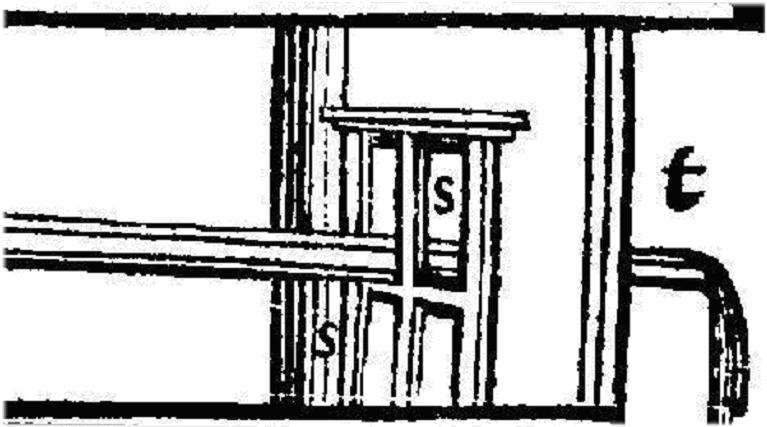
There are three parts labelled thus but owing to the complete list of lettered items in the parts list, one might assume that the letter ‘W’ was intended, except that the the letters ‘u/v’ which one would think should follow the last letter shown - ‘t’ - appear to be missing. Why then is the last item called item 10? The solution seems obvious; the intention is that the reader should replace all the letters with numbers. The letters are found to run from ‘a’ to ‘t’, plus the letter ‘10’. ‘10’ is the last item on the list and if it does not representt the letter ‘u/v’ then perhaps it represents the letter ‘j’? The letter ‘j’ is omitted from the list of parts and does not appear in the drawing, but ‘j’ is an alternative to the letter ‘i’, which does appear. The letter ‘j’ is the tenth letter of the English alphabet so it may have been Bessler’s intention to convey that idea of using the letter ‘j’ as the tenth letter for the reasons that follow.

I mentioned that there seemed the usual over-use of numbers per parts and an example is the use of the letter ‘s’, see the enlarged example below.  In this case the letters are very, very small and very close to each other and therefore could be regarded as superfluous. This enlargement is taken from the bottom right of the main drawing above.

This is just one example of many. This is what leads one to conclude that a specific total is being sought. The letters transcribed into numbers results as follows:-

1,1,2,2,2,3,3,3,4,4,5,6,6,7,7,7,7,8,8,8,9,9,10,10,10,11,11,12,12,13,14,15,16,16,17,18,
19,19,20.

I have inserted the number 10 and assumed that it stands for the letter ‘j’ in which case all the letters following ‘j’ are advanced by one, in their alphanumeric code. I should point out here, that in other examples of alphanumeric coding by Bessler he has appeared to use the standard ‘j/i’ and ‘u/v’ alternatives rather than requiring us to add the ‘j’ as a separate letter in its own right and therefore we should bear this in mind when attempting to decipher other clues.

There are 39 numbers totalling 355. Knowing Bessler’s fondness for pentagonal numbers,, one must conclude that there is one number missing and that it is the number 5, (it’s that number 5 again!) and this brings the quantity of numbers used up to 40 - and the total to 360. There are 20 letters used, including the letter ‘j’, and 20 letter/numbers in the left half of the drawing, and 19 in the right half, but with the missing number 5 to add to the 19 it makes 20 in each half, so 360 divided by 20 = 18, the pentagonal number again - of course 360 divided by the missing 5 equals 72.

There is more to the use of the letter ‘j’ than simply hinting that there could be an alphanumeric substitution possible. ‘J’ is the tenth letter of the alphabet and was omitted. In the atbash cipher the letter ‘J’, when unattached to its alternative letter ‘i’, is equal to ‘W’, which strangely enough is what the coded ‘J/10’ looks like. This suggests that there may be an example of the atbash cipher contained within the picture too. In passing, it is worth pointing out that ‘W’ also represents 55 in Bessler’s code as two ‘v’s as shown in the ‘W’ of Weissenstein above the right hand picture.(see also Bessler’s handwritten ‘W’s)

There are other clues buried in this illustration. For instance in the picture below one can see four red lines which I have drawn in, connecting the ‘10’s. There are several angles introduced which confirm the intention to promote interest in the pentagram.

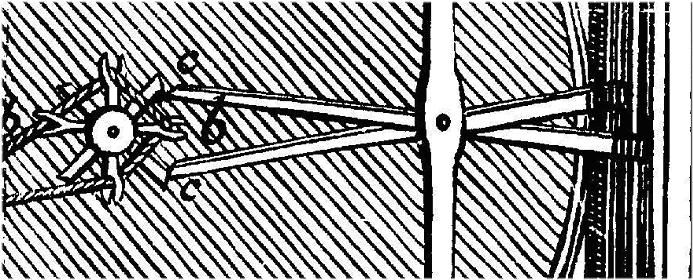
The blue horizontal line joins the two ‘p’s with the upper ‘d’. The vertical green line joins the upper remaing ‘10’ with the point where the diagonal from the adjacent ‘10’ crosses the bottom line of the drawing. Notice that in the right drawing the red line starting from the bottom left rises to meet the letter ‘d’ at the same point as the blue horizontal line. It also parallels one of the ropes. There are some more hints at pentagrams but I think that is enough for now. There is another feature which is interesting and definitely deliberate.

I was examining the details of the drawing itself, and noticed that on the right side of the right picture there is an upright supporting beam which carries two rods. These connect the ‘spokes’ on the axle of the wheel with the stampers and seem designed to lift them as the wheel rotates. The odd thing about the design is that on the left side of the upright the upper rod is outside the lower one, or nearer to the viewer and yet on the right side of the beam, the same rod, now the lower one is inside the upper one, or further from the viewer. You need to study the design to see what I mean but I have included an enlargement of this feature from the main drawing below, so that you can understand the point. On the face of it, the rods must be bent at the middle in order to achieve this effect – or, the rods are not connected right through to the other side of the beam. The reason for this could be that we are intended to ignore the right side of the beam, or that they are simply bent, but for no apparent reason other than whim. Examination of the right hand end of the two rods also reveals that the rods have been interchanged where they intrude into the vertical stampers. 

There is more to discover in this drawing but you will need other drawings for comparison purposes and I do not wish to devote any further discussion of this drawing here. Having said this I am likel;y tomake alterations and additions from time to time as and when the information is learned.

Copyright © 2011 John Collins.